Transcript Ex 131, 22 lim┬ (x → π/2) tan2x/ (x − π/2) lim┬ (x → π/2) tan2x/ (x − π/2) Putting y = x – π/2 When x → 𝜋/2 y → 𝜋/2 – 𝜋/2 y → 0 So, our equation becomes lim┬ (x→π/2) tan2x/ (x − π/2) = lim┬ (y→0) (tan2 (𝜋/2 𝑦)/𝑦) = lim┬ (y→0) ( (〖tan 〗〖 (𝜋 2𝑦〗))/𝑦) = lim┬ (y→0) (tan2𝑦/𝑦) = lim┬ (y→0) (1/𝑦 tanx = sinx cosx For x = π 2 the denominator of this expression is zero, so it cannot be calculated Answer link Alan P tan( π 2) is undefinedTo find tan 2x We know that, tan 2x = 2 tanx 1−tan2x 2 t a n x 1 − t a n 2 x Putting the values, we get tan 2x = − 1 119 − 1 119

If Tan X 512 And Pi2 X Pi Find The Values Of Tan 2x
What is the value of tan pi by 2
What is the value of tan pi by 2-//drivegooglecom/file/d/1jztwNgaiCLZYj6WslAR8EVssDylKkid/view?usp=drivesdk11th STD BUSINESS MATHSTAMILNADU SYLLABUSCHAPTER 4 TRIGONOMETRYSUBSCRIBE SUse our tan(x) calculator to find the tangent of π/2 radian(s) tan(π/2 rad) or the tangent of any angle in degrees and in radians To use this calculator, just type a value for the angle, then press 'Calculate' You may choose radians (rad) or degrees (°) as the angle unit



Consider The Function Below F X 8x Tan X Pi 2 Chegg Com
Find the value of the expression sin(2 tan1(/3) cos(tan12√2) asked in Class XII Maths by rahul152 ( 2,8 points) inverse trigonometric functions Ex 22, 17 Find the values of tan1(tan〖3π/4〗 ) Let y = tan1(tan〖3π/4〗 ) tan y =〖 tan〗〖3π/4〗 tan y = tan (135°) Since Range of of tan1 is (− 𝜋/2 , 𝜋/2 ) ie (− 90° ,90°) Hence, y = 135° not possible Now, tan y = tan (135°) tan y = tan (180° – 45°) tan y = – tan (45°By definition tan is sin/cos and since the sin of pi/2 is 1 and the cos of pi/2 is 0 the tan of pi/2 is an undefined value, because you cannot divide anything by zero
If 3 tan1 x cot1 x = π, then x equals (a) 0 (b) 1 (c) 1 (d) 1/2 Questions from AIIMS 10 1 An alternating current i in an inductance coil varies with time according toClick here👆to get an answer to your question ️ lf x ∈( pi/2,pi/2) , then the value of tan^1 (tanx/4) tan^1 (3sin2x/5 3cos2x ) is Join / Login biology lf x ∈ (− 2 π , 2 π ), then the value of tanThe field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies The Greeks focused on the calculation of chords, while mathematicians in India created the earliestknown tables of values for trigonometric ratios (also called trigonometric functions) such as sine
Use our tan(x) calculator to find the tangent of π/2 radian(s) tan(pi/2 rad) or the tangent of any angle in degrees and in radians To use this calculator, just type a value for the angle, then press 'Calculate' You may choose radians (rad) or degrees (°) as the angle unitSum or Difference of angles Rd Sharma Class 11 chapter wise VSAQ solutions Ex 21, 6 (Method 1) Find the principal value of tan−1 (−1) Let y = tan−1 (−1) y = − tan−1 (1) y = − 𝝅/𝟒 Since Range of tan−1 is (−π/2,π/2) Hence, Principal Value of is (−𝝅)/𝟒 We know that tan−1 (−x) = − tan −1 x Since tan 𝜋/4 = 1 𝜋/4 = tan−1 (1) Ex 21, 6 (Me




If Tan Pi 4 Theta Tan Pi 4 Theta 3 Then The Value Of Tan 2 Pi 4 Theta Tan 2 Pi 4 Theta



How To Evaluate Tan 2 Tan 1 1 5 Pi By 4 Quora
Find the value of tan−1−1√3cot−11√3tan−1sin−π2 We have tan−1−1√3cot−11√3tan−1sin−π2=tan−1tan5π6cot−1cotπ3tan−1−1=tan−1tanπ−π6cot−1cotπ3 ⎡ ⎢ ⎢ ⎢ ⎣ ∵ t a n − 1 (t a n x) = x, x ∈ (− π 2α β = π/2 and β γ = α Thus, γ = α – β Taking "tan" on both sides, tan γ = tan (α – β)Free tangent line calculator find the equation of the tangent line given a point or the intercept stepbystep This website uses cookies to ensure you get the best experience By using this website, Simple Interest Compound Interest Present Value Future Value Conversions




Ex 3 3 7 Prove Tan Pi 4 X Tan Pi 4 X 1 Tan X 2



If Tan Pi Cos Theta Cot Pi Sin Theta Then Prove Cos Theta Pi 4 1 2 Sqrt2 Youtube
VITEEE 12 The value of limx→∞((π/2) tan1 x)1/x is (A) 0 (B) 1 1 (D) e Check Answer and Solution for above Mathematics question Tardigrade Example 28 If tan𝑥 = 3/4 , "π" < 𝑥 < 3𝜋/4 , find the value of sin 𝑥/2 , cos 𝑥/2 and tan 𝑥/2 Given that "π" < x < 3𝜋/2 ie180° < x < 3/2 × 180° ie 180° < x < 270° Dividing by 2 all sides (180°)/2 < 𝑥/2 < (270°)/2 90° < 𝑥/2 < 135° So, 𝑥/2 lies in 2nd quadrant In 2nd quadraTan π/2 Value with solutions Mathematics Answer Comment 2 answers Georgia 21 2 months ago 4 0 Tan(x)=sin(x)/cos(x) so tan(pi/2)=1/0, which is undefined Send Rina 55 2 months ago 3 0 Answer Stepbystep explanation The given expression is We know that the tangent function is defined as the quotient between the sin and cosin




2 Pi 2 R The Value Of Tan 2



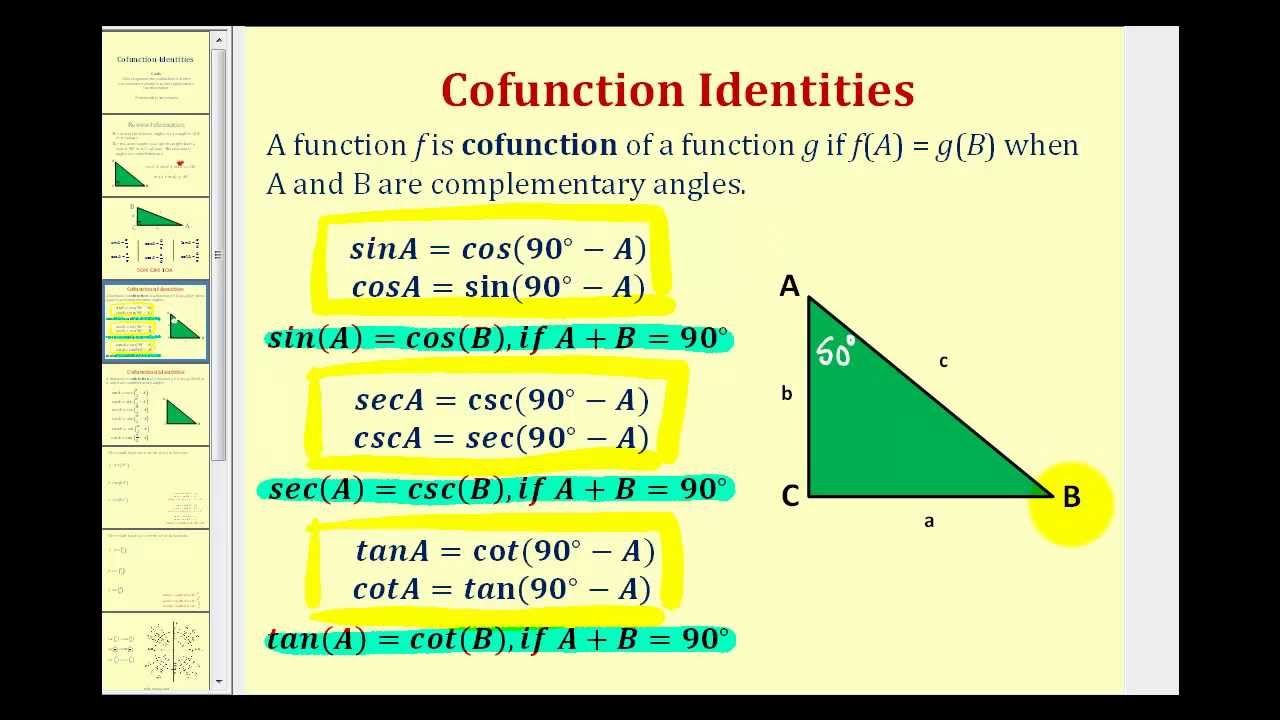
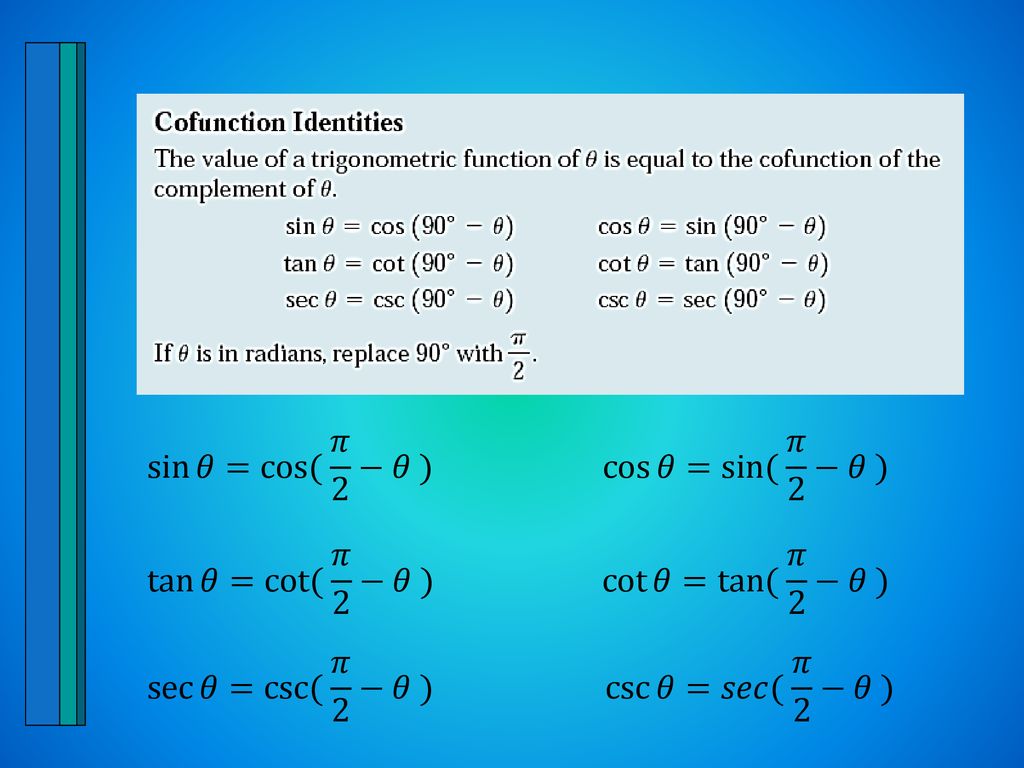
Co Functions Solutions Examples Videos
Use our tan(x) calculator to find the tangent of π/2 radian(s) tan(π/2 rad) or the tangent of any angle in degrees and in radians To use this calculator, just type a value for the angle, then press 'Calculate' You may choose radians (rad) or degrees (°) as the angle unitWhen we find sin cos and tan values for a triangle, we usually consider these angles 0°, 30°, 45°, 60° and 90° It is easy to memorise the values for these certain angles The trigonometric values are about the knowledge of standard angles for a given triangle as per the trigonometric ratios (sine, cosine, tangent, cotangent, secant and cosecant)Tan−1(sin( 2−π )) = tan−1(−sin(2π )) = tan−1(−1) =−4π



Mfg The Tangent Function And Cofunctions
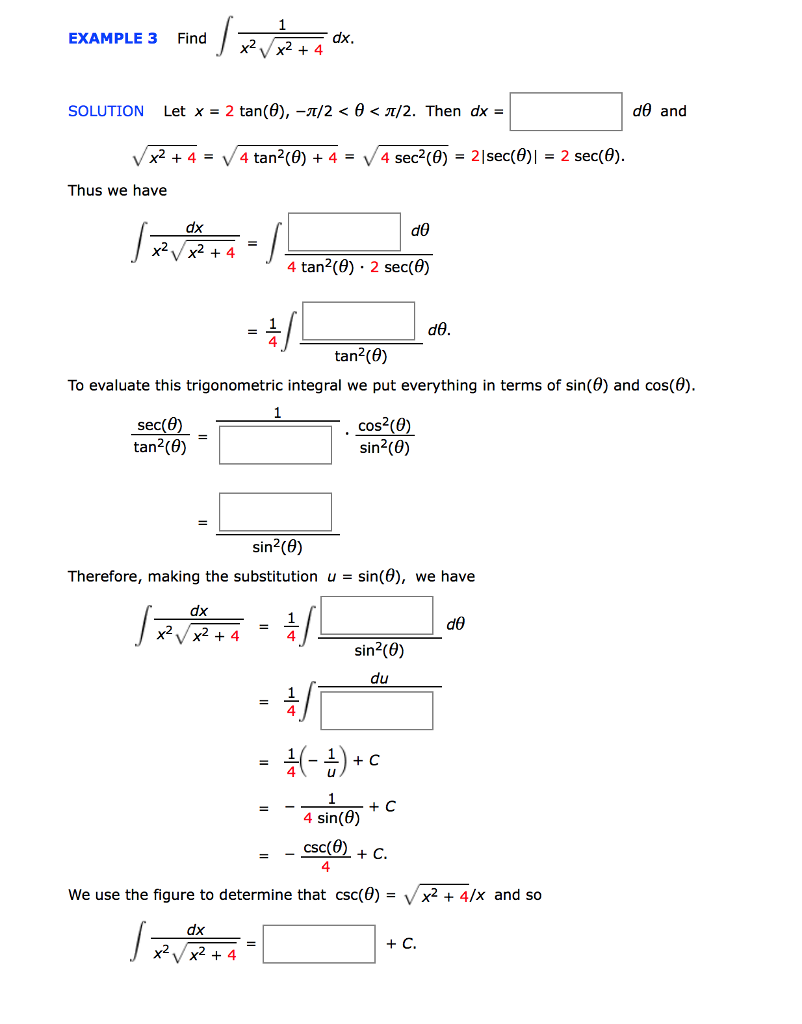



Example 3 Find Dx Solution Let X 2 Tan 8 Z 2 Chegg Com
If cot α = 1/2, sec β = 5/3, where π < α < 3π/2 and π/2 < β < π, find the value of tan(α β) State the quadrant in which α β terminatesThe value of ∫_ (π/2)^ (π/2)dx/ ( x sinx 4) where t denotes the greatest integer less than or The value of ∫_ (π/2)^ (π/2)dx/ ( x sinx 4) where t denotesAnna from SVSU Micro Math helps you evaluate tangent of a number by using the unit circle The tangent value in this problem is undefinedProblem Find tan
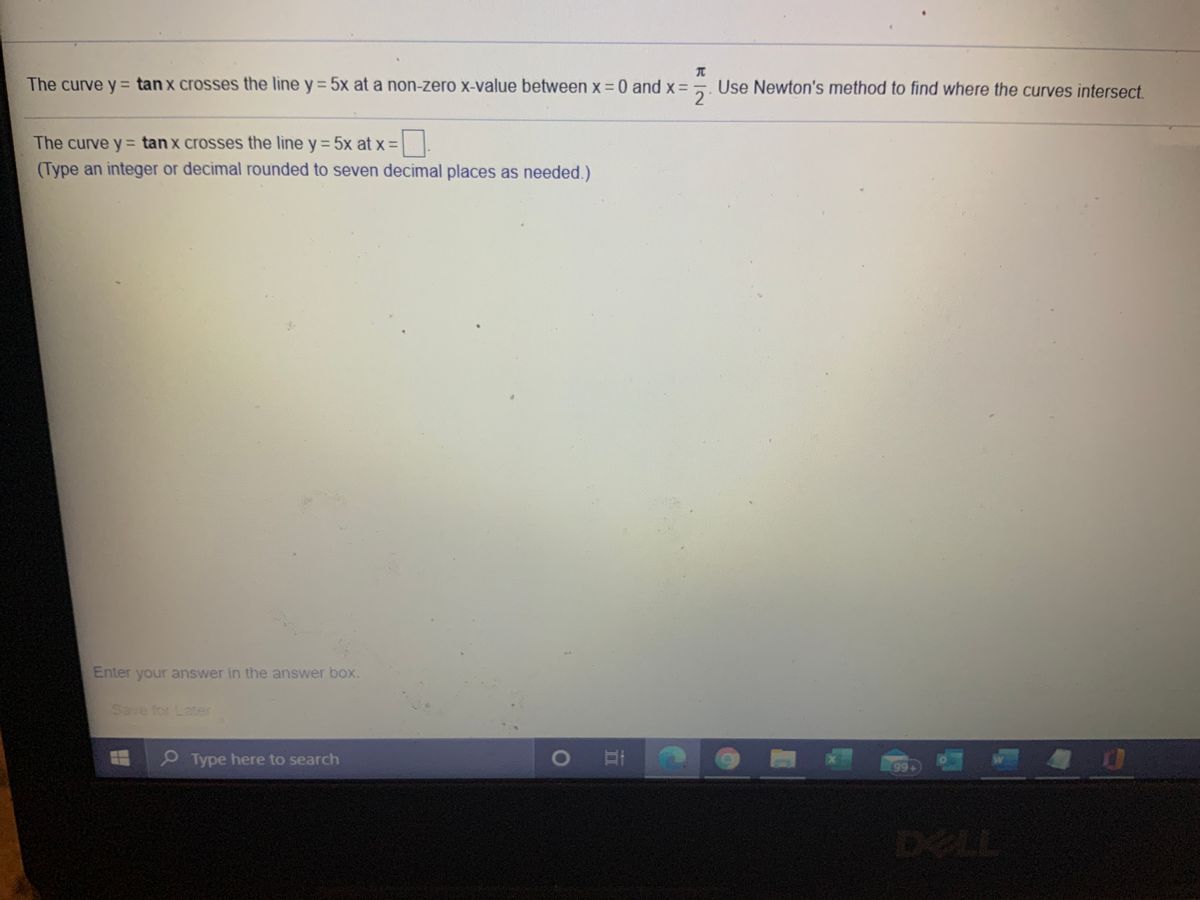



Answered The Curve Y Tan X Crosses The Line Y Bartleby
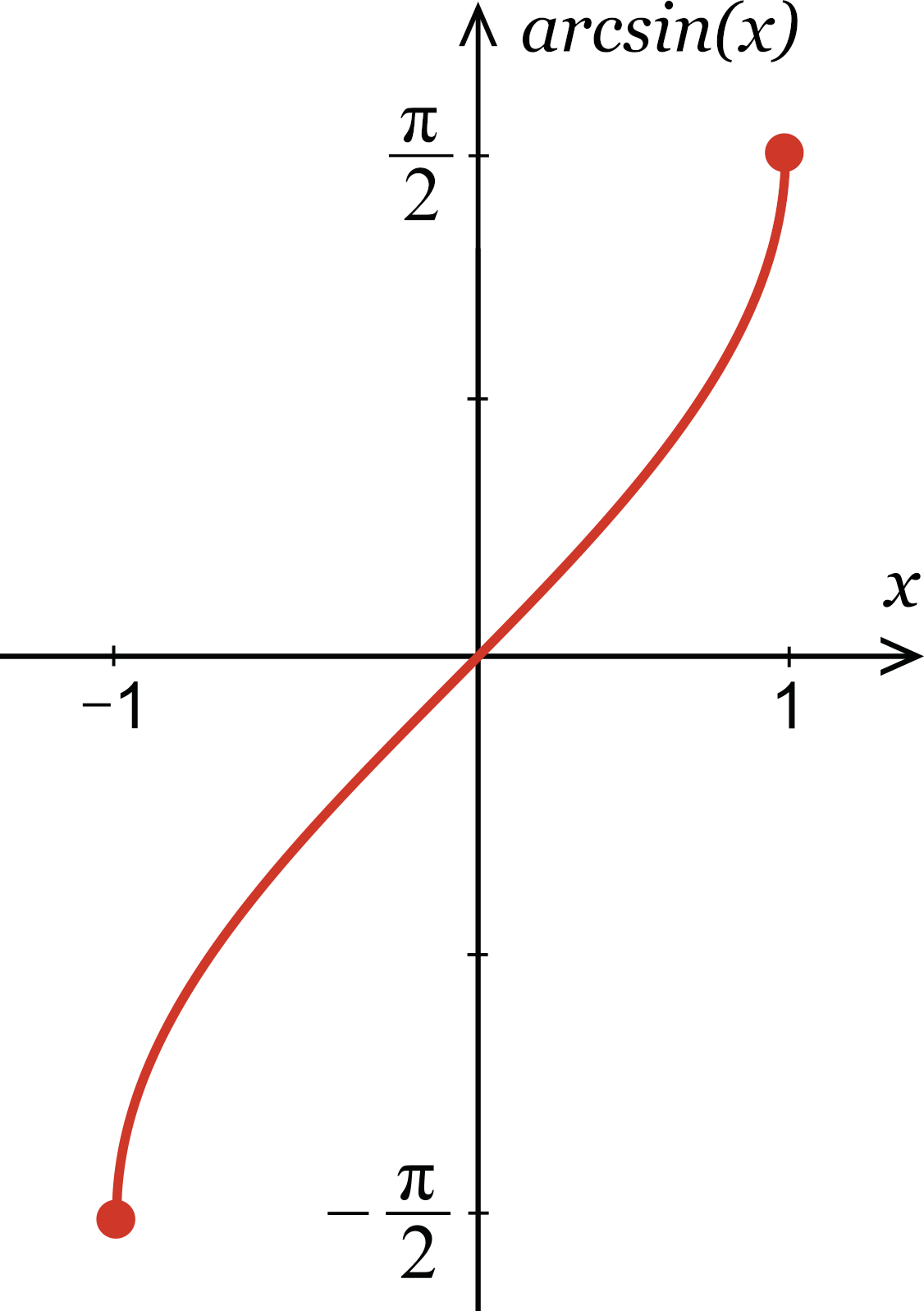



Inverse Sine Calculator Calculate Arcsin X Inch Calculator
If sin A = 1/2, cos B = 12/13, where π/2 < A < π and 3π/2 < B < 2π, find tan(A – B)Tan(π/2) ① Find the value of the trigonometric function (sin(π/2))/(cos(π/2)) ② Find the value of the trigonometric function 1/(cos(π/2)) ③ Find the value Best answer tan1 sin (π/2) = π/4 Detailed Answer Please log inor registerto add a comment ← Prev QuestionNext Question → Related questions 0votes 1answer Principal value of tan^ 1 1 cos^1 (1/2) sin^1(1/2) is equal to (a)2π/3 (b)3π/4 (c)π/2 (d) 6π




Consider The Function Below F X 8x Tan X Pi 2 Chegg Com




If Tan X 512 And Pi2 X Pi Find The Values Of Tan 2x
A quarter turn, or 90°, or π / 2 radian is a halfperiod shift for tan(x) and cot(x) with period π (180°), yielding the function value of applying the complementary function to the unshifted argument By the argument above this also holds for a shift by any odd multiple (2k 1)⋅ π / 2 of the half period You have no way to assign a value to tan(π/2) so that its value is compatible with usual algebraic rules together with the trigonometric identities Taking into account why is tan PI 2 Infinity?In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a rightangled triangle to ratios of two side lengths They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others




42 Printable Unit Circle Charts Diagrams Sin Cos Tan Cot Etc




The Expression Tan X Pi 2 Cos 3pi 2 X Sin 3 7pi 2 X Cos X Pi 2 Tan 3pi 2 X Simplifies To A Brainly In
Trigonometry Find the Exact Value tan (pi/2) tan ( π 2) tan ( π 2) Rewrite tan( π 2) tan ( π 2) in terms of sines and cosines sin(π 2) cos(π 2) sin ( π 2) cos ( π 2) The exact value of cos(π 2) cos ( π 2) is 0 0 sin(π 2) 0 sin ( π 2) 0 The expression contains a division by 0 0 The expression is undefined UndefinedIf we view tan as function from the usual real line to the projective real line, then we can continuously extend it so that tan ( π / 2) = ∞ It depends on how you interpret the situation π 2 = 1 0 which is undefined in mathematics ( x) = ∞ Both cases are equally correct and valid Prove (i) 2tan^1 (3/4) – tan^1 (17/31) = π/4 (ii) 2tan^1 (1/2) tan^1 (1/7) = tan^1 (31/17)




Given Sin 8 3 5 And 0 8 P 2 Find The Exact Value Of The Function Sin 8 2 Wyzant Ask An Expert



Http Functions Wolfram Com Introductions Pdf Tan Pdf
Tangent begins its period at , finishes it at , and then repeats it (forward) over to Cotangent begins its period at 2 π k , {\displaystyle 2\pi k,} finishes it at 2 π k π , {\displaystyle 2\pi k\pi ,} and then repeats it (forward) over 2 π k π {\displaystyle 2\pi k\pi } to 2 π k 2 π Inverse Trigonometric Formulas Trigonometry is a part of geometry, where we learn about the relationships between angles and sides of a rightangled triangleIn Class 11 and 12 Maths syllabus, you will come across a list of trigonometry formulas, based on the functions and ratios such as, sin, cos and tanSimilarly, we have learned about inverse trigonometry concepts alsoTan π/2 Value with solutions Sign up NOw and post your Question Assignment Grade Search Search Post Unanswered Categories LOGIN JOIN US Post a Question Tan π/2 Value with solutions Mathematics Posted by admin QUESTION POSTED AT 1146 PM Answered by admin AT 1146 PM




Pi In R Tutorial With Example




Check My Work Evaluating Tan Frac 7 Pi 8 Using A Half Angle Formula Mathematics Stack Exchange
The value of lim x→2 5 / √2 √x is The value of lim x→π/2 sin (cos x) (cos x) / sin x cosec x is The Value Of N For Which 1 Yn 1 Yn Is The Geometric Mean Of X And Y Is The value of power dissipated across the Zener diode (V z = 15 V) connected in the circuit as shown in the figure is x*10 –1 watt The value of sinClick here👆to get an answer to your question ️ If tan (picos theta) = cot (pi sintheta) than a value of cos ( theta pi/4 ) among the following is FacebookWhatsapp Transcript Ex 21, 14 (Method 1)Find the value of tan−1 √3 – sec−1(–2) is equal to π (B) – π/3 π/3 (D) 2π/3Solving tan−1 √𝟑Let y = tan−1 √3tan y = √3 tan y = tan (𝝅/𝟑)∴ y = 𝝅/𝟑 Since Range of tan−1 is ((−𝜋)/2,𝜋/2)Hence, principal value is



Solved If U Log Tan Pi 4 Theta 2 Course Hero
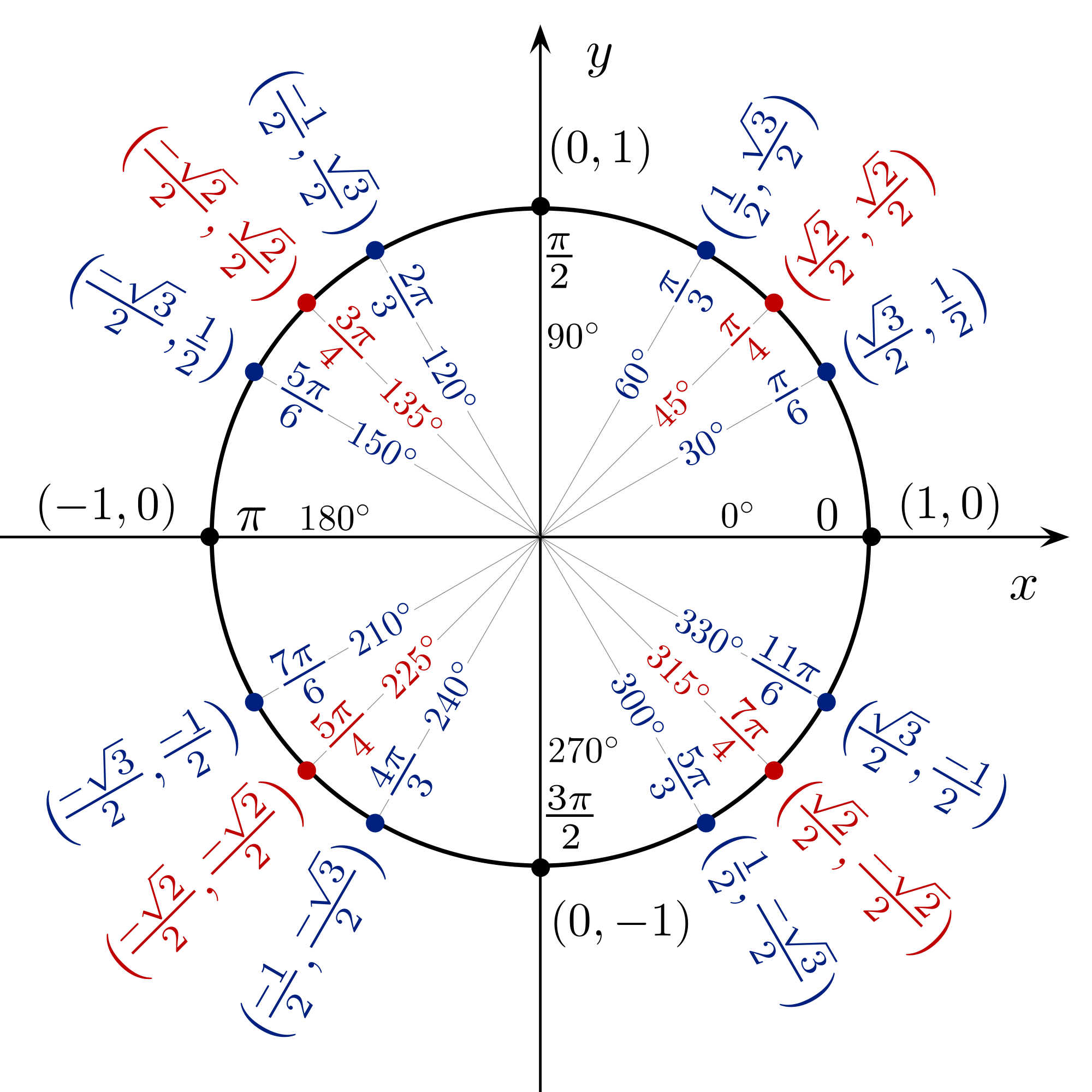



How Do You Find The Sine Cosine And Tangent Of 19pi 2 Radians Socratic
Correct answer If tan x = 3/4, π< x< 3π/2 ,find the value of sin x/2, cos x/2 ,tan x/2 eanswersincomSolution Given, tan x = 3/4 , π < x < 3π/2 ⇒ π/2 < x/2 < 3π/4 (belongs to quadrant II) In the second quadrant, cos (x/2) is negative sec 2 x = 1 tan 2 x = 1 (3/4)2 = (16 9)/16 = 25/16 sec x = ± 5/4 Thus, cos x = 4/5 {since π < x < 3π/2} Using the formula, cos 2A = 2 cos 2 A – 1,




Example 27 Find Value Of Tan Pi 8 Chapter 3 Class 11




Rd Sharma Solutions For Class 11 Chapter 7 Values Of Trigonometric Functions At Sum Or Difference Of Angles



Section 4 3 Right Triangle Trigonometry Precalculus



Pplato Flap Math 1 6 Trigonometric Functions




Domain Range Of Inverse Tangent Function Video Khan Academy




The Value Of Tan Pi 16 2tan Pi 8 4 Is Equal To Youtube




Tan Pi 4 Theta Tan Pi 4 Theta 2tan2theta Youtube




Cos P A Cos P 2 A Cos A Tan P A Tan 3p 2 A Sin 2p A Cosa Brainly In



Right Triangle Trigonometry Ppt Download




Given Tan 8 8 Where Frac 1 P 2 8 Subse Gauthmath




Find The Exact Value Of Tan 2 Pi 16 Tan 2 3pi 16 Tan 2 5pi 16 Tan 2 7pi 16 Youtube




Find The Exact Value Of The Trigonometric Expression Given That Sin U 3 5 Where 3p 2 U 2p And Cos V 15 17 Where 0 V P 2 Tan U V Wyzant Ask An Expert




The Value Of 2 Tan Pi 10 3 Sec Pi 10 4 Cos Pi 10 Is Equal A 0 B Askiitians




Ex 13 1 22 Lim X Pi 2 Tan 2x X Pi 2 Chapter 13 Class 11




Aman Siwach The Value Of Cosec 1 X 2x Is A X B P 2 Tan X C 2tan X D None Of These T Co Hg2cejg54s Via Pinterest Math Problem Trigo Trigonometry Study T Co Dqa9qw7khs




Find The Principal Value Of Tan 1 Sin Pi 2




General Solution Of Tantheta Tan Pi 2 Theta 2 Is




Prove These Cot Pi By 8 Minus Tan Pi By 8 Is Equal To 2 Brainly In
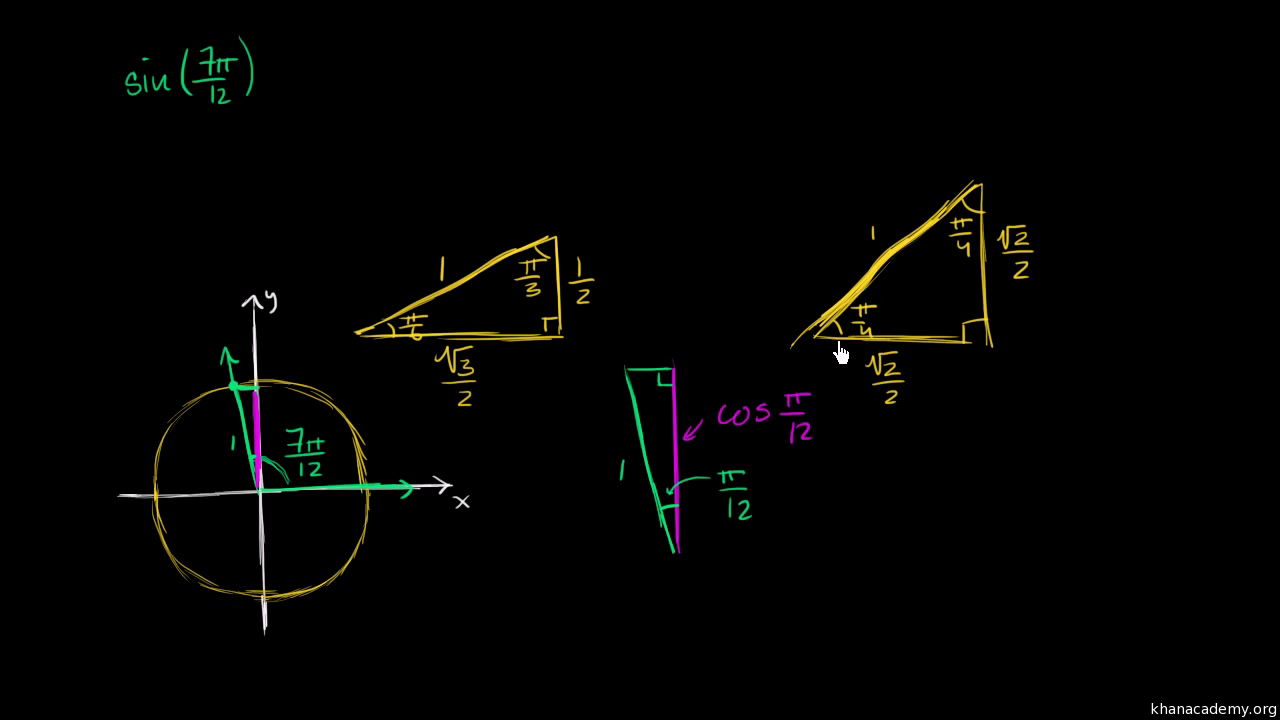



Finding Trig Values Using Angle Addition Identities Video Khan Academy




If Tan 1 X Sin 1 X Pi 2 Prove That 2x 2 1 Root 5 Maths Inverse Trigonometric Functions Meritnation Com
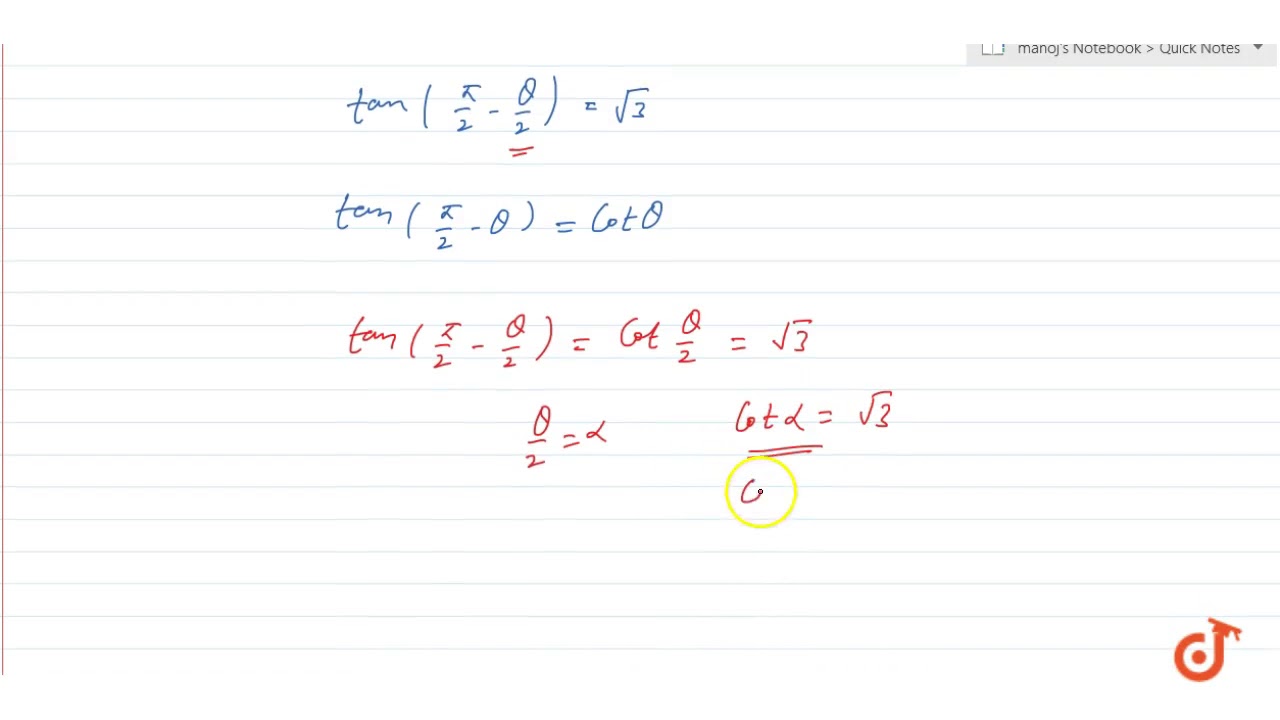



If Tan Pi 2 Theta 2 Sqrt 3 The Value Of Cos Theta Is Youtube




How Do You Determine The Exact Value For Cos Pi 6 Csc Pi 3 Sin Pi 4 Socratic
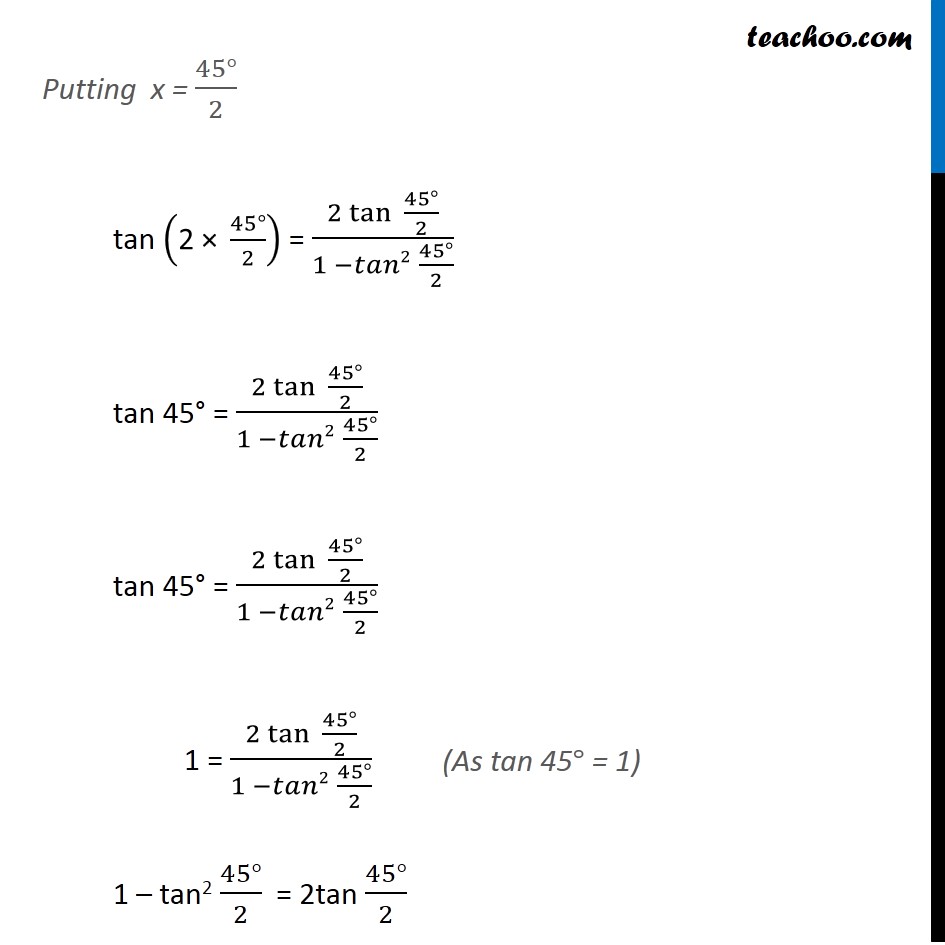



Example 27 Find Value Of Tan Pi 8 Chapter 3 Class 11




Limits Of Trigonometric Functions Video Khan Academy




Cosa 1 Sina Tan P 4 A 2 Brainly In




Prove That Tan Pi2 X Sec Pi X Sin X Sin Pi X Cot 2pi X Pi2 X 1
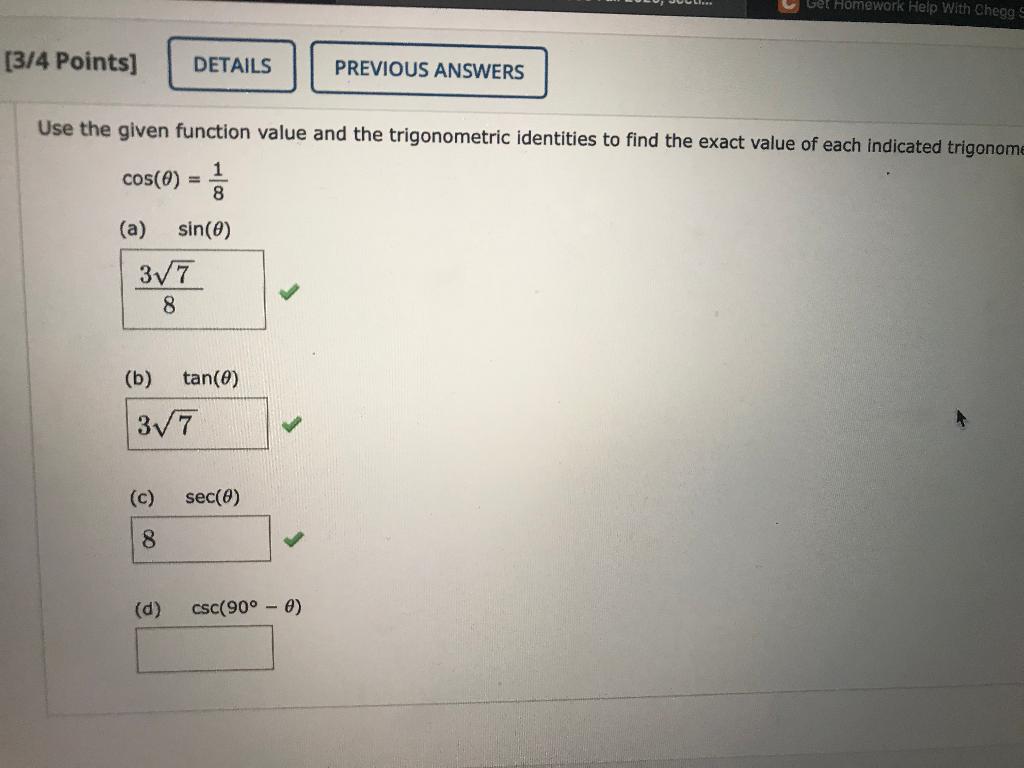



Use The Given Function Value And The Trigonometric Chegg Com



Web Auburn Edu Holmerr 1617 Textbook Trigonometry Print Pdf




2 Tan 2 P 4 3 Sin 2 P 3 3 Cos 2 P 6 Trigonometric Values Sci Pi



1



1




File Mplwp Sin Cos Tan Piaxis Svg Wikimedia Commons



Http Www Math Miami Edu Dscheib Teaching Fall09 Mac1147 Lecture Notes Files Section 7 2 Notes Pdf




Prove That Sec Theta Tan Theta Tan Pi 4 Theta 2




The Value Of I Y Cos Square Root Of Sin Tan P Gauthmath
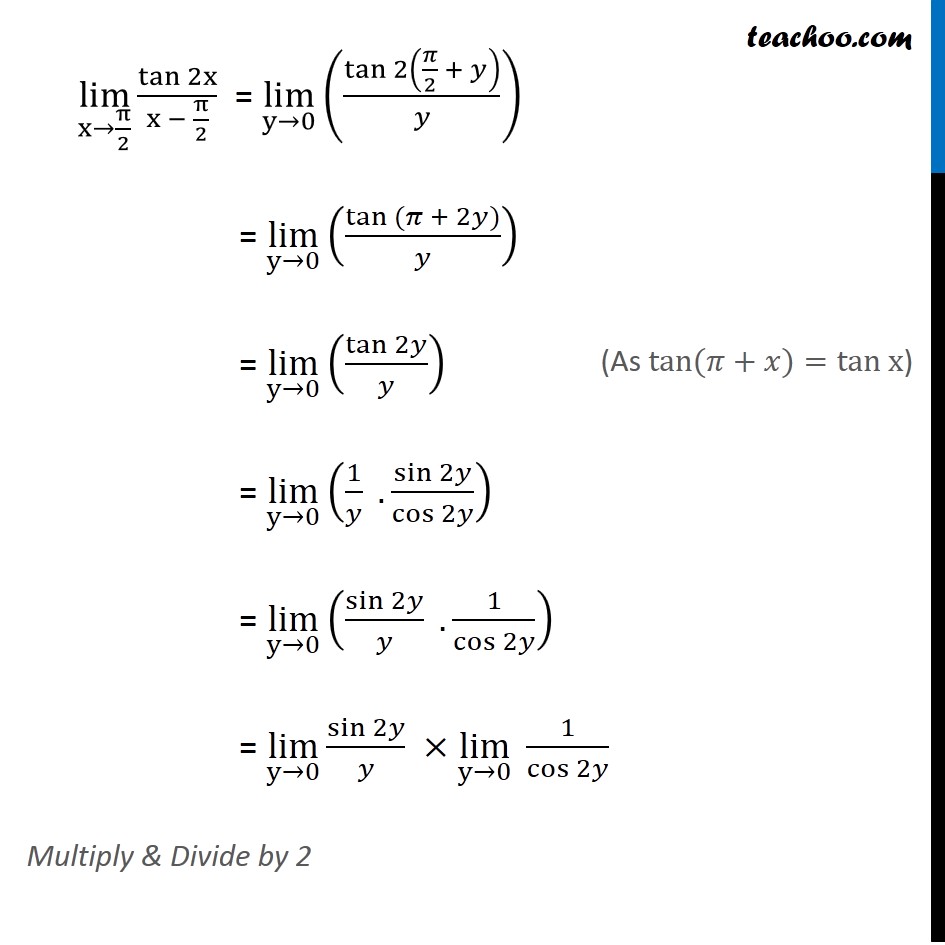



Ex 13 1 22 Lim X Pi 2 Tan 2x X Pi 2 Chapter 13 Class 11




Tan Pi 4 Theta Tan Pi 4 Theta 2sec2theta 1 2si2o1 Tan 0 Tan 4 0 2 Sec Youtube
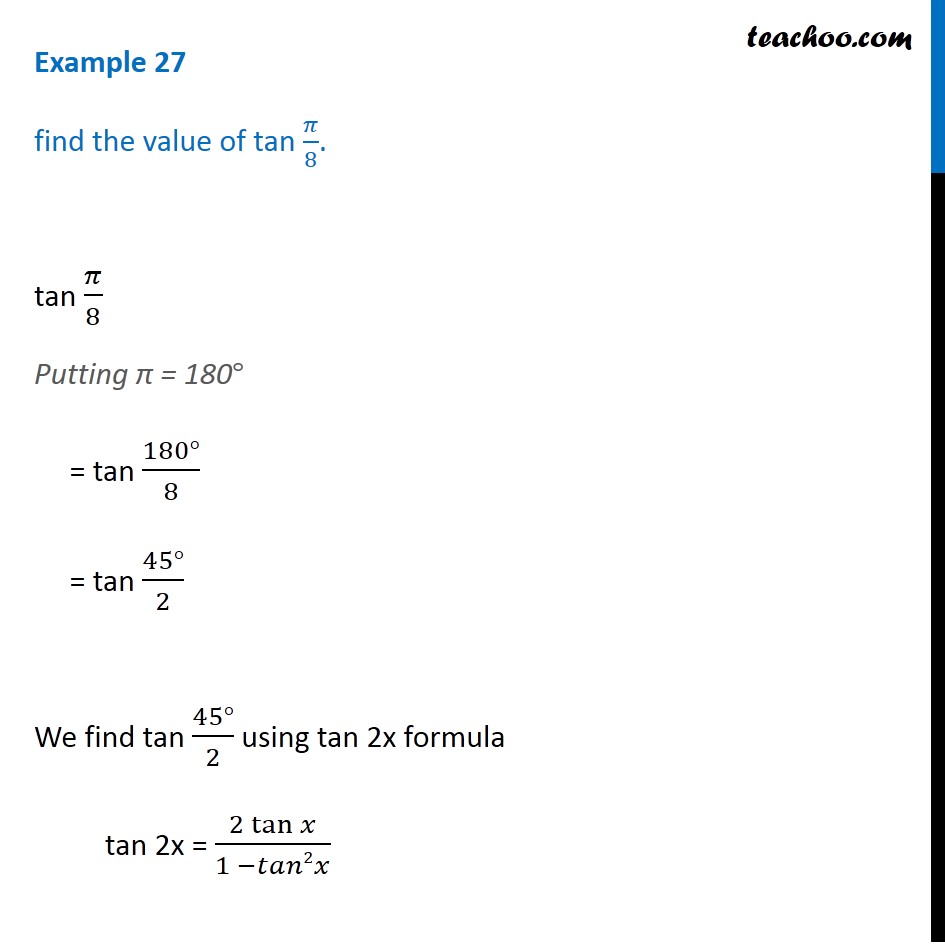



Example 27 Find Value Of Tan Pi 8 Chapter 3 Class 11
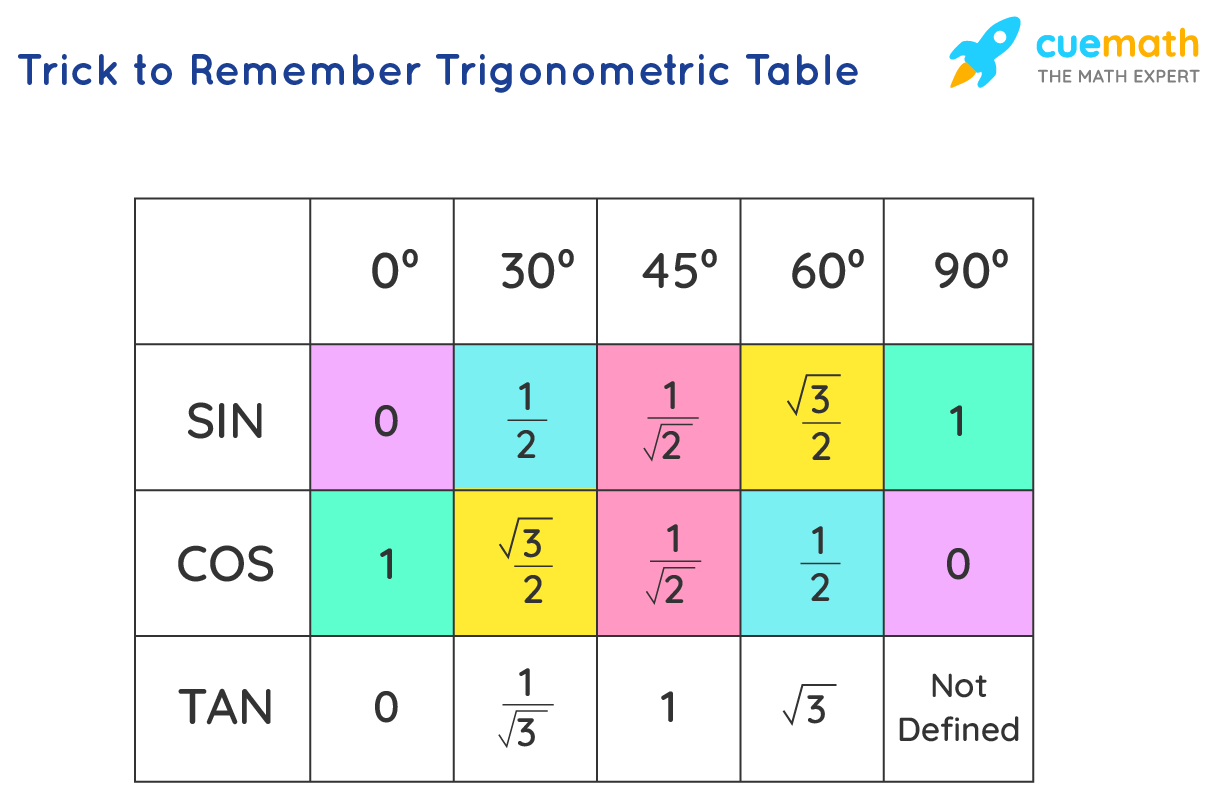



Trigonometric Table Formula Trigonometry Ratio Table



Users Math Msu Edu Users Causleym Hw1 Solutions Pdf



What Is Lim X Pi 2 1 Sinx Tan X 2 1 Quora



Ppt Right Triangle Trigonometry Powerpoint Presentation Free Download Id




How Do I Find The Value Of Tan 3pi 4 Socratic




Tan 18 Proof Tan P 10 Proof
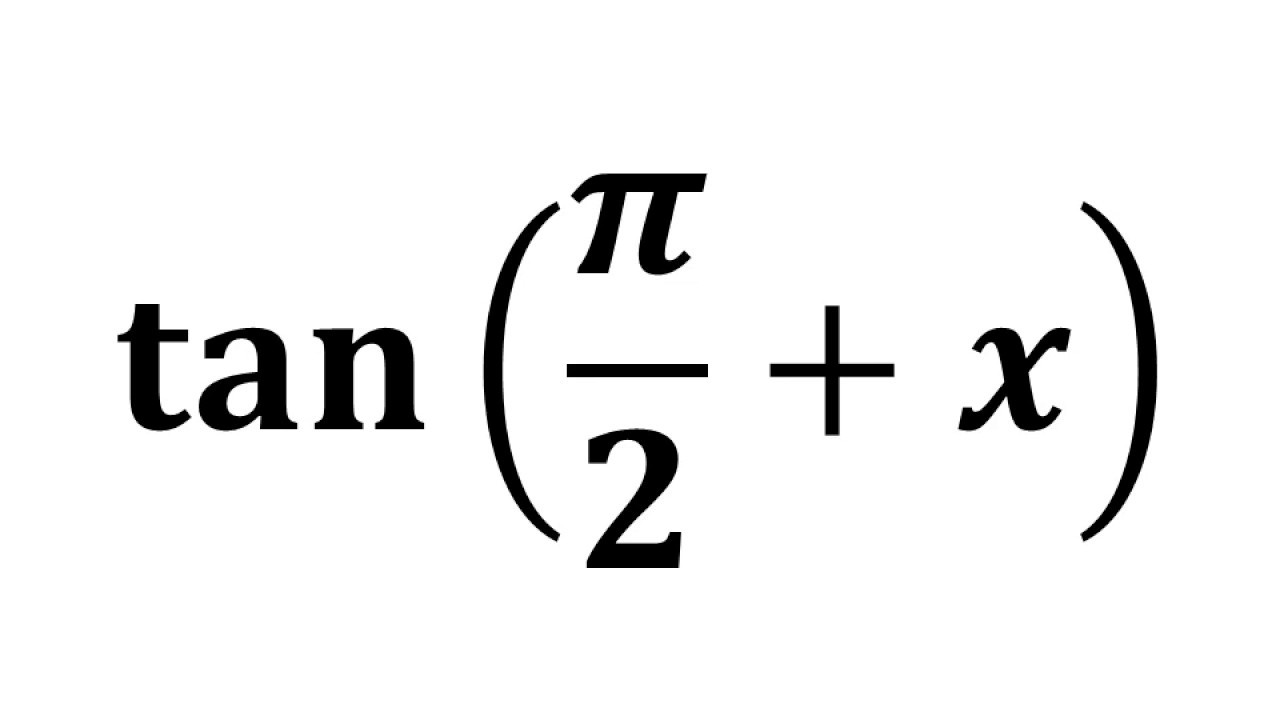



Tan Pi 2 X Tan Pi 2 Theta Youtube




Trigonometric Functions Angles Tables Degree Radians Sin Cos Tan
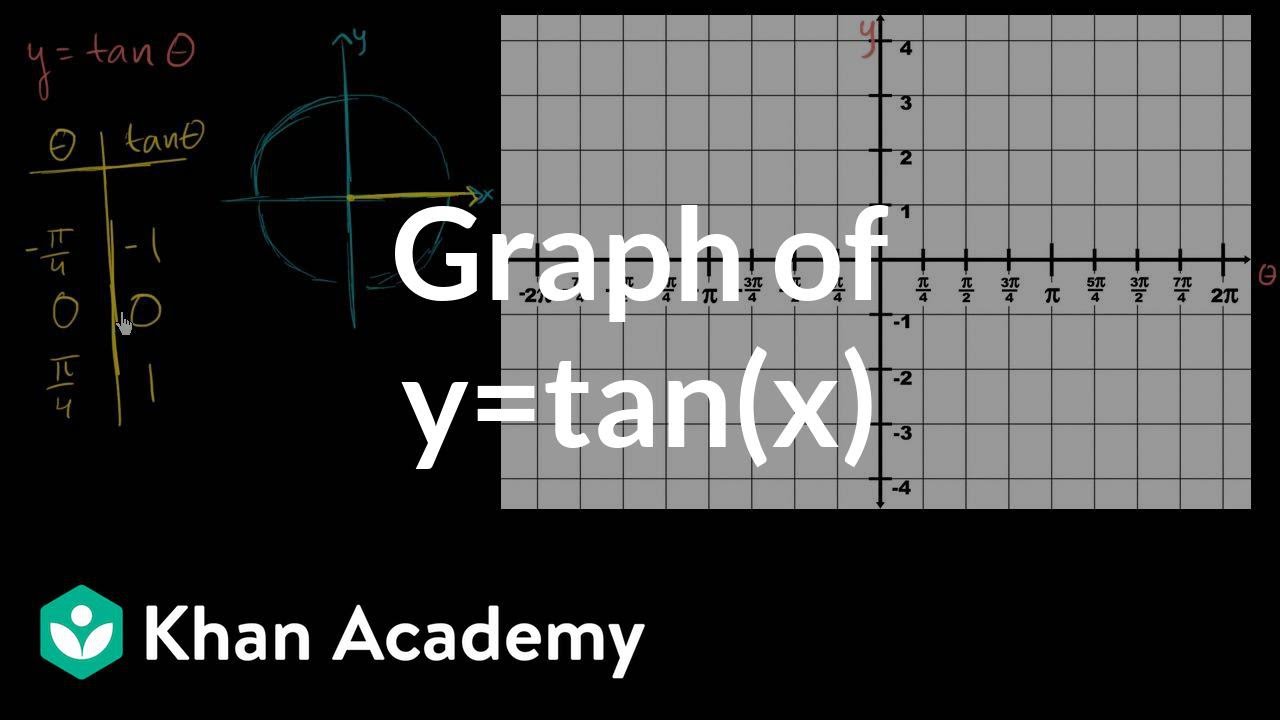



Graph Of Y Tan X Video Trigonometry Khan Academy




Prove That Sin Pi X Cos Pi2 X Tan 3pi2 X Cot 2pi X Sin 2pi X Cos 2pi X X Sin 3pi2 X N Pi Pi 4 Where N N




Value Of Tan Pi 2 Mathematics Stack Exchange




Select All That Have A Value Of 0 Cos Frac P 2 Gauthmath




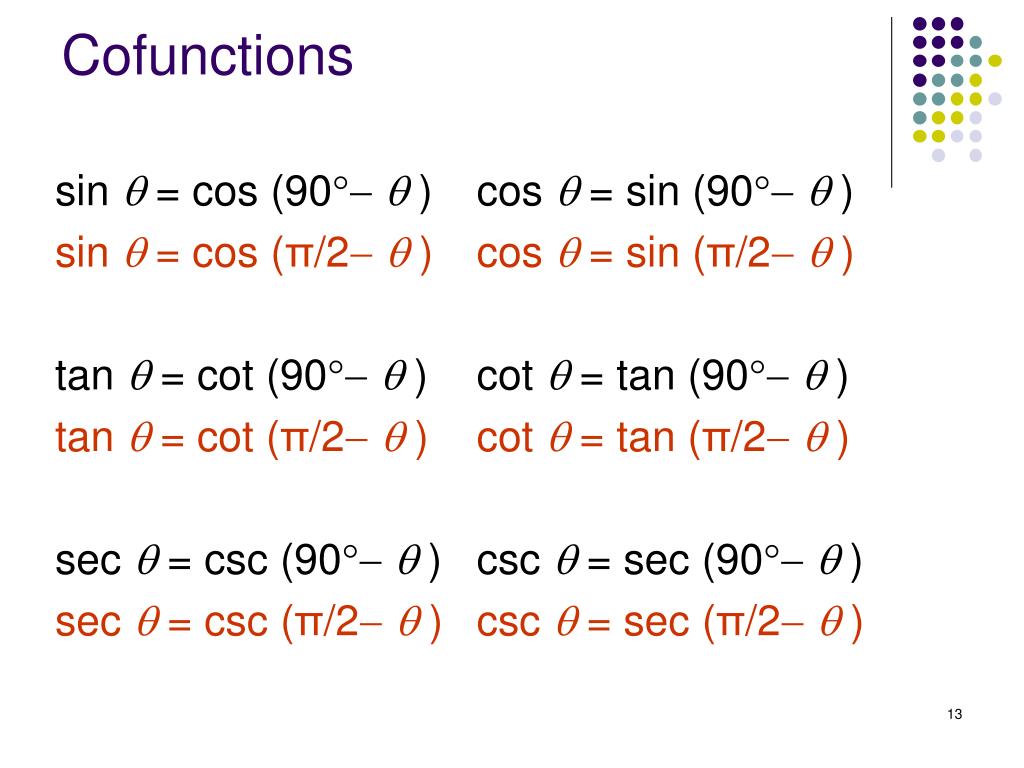
Cofunction Identities In Trigonometry With Proof And Examples Owlcation




Find The Value Of Tan Pi 16
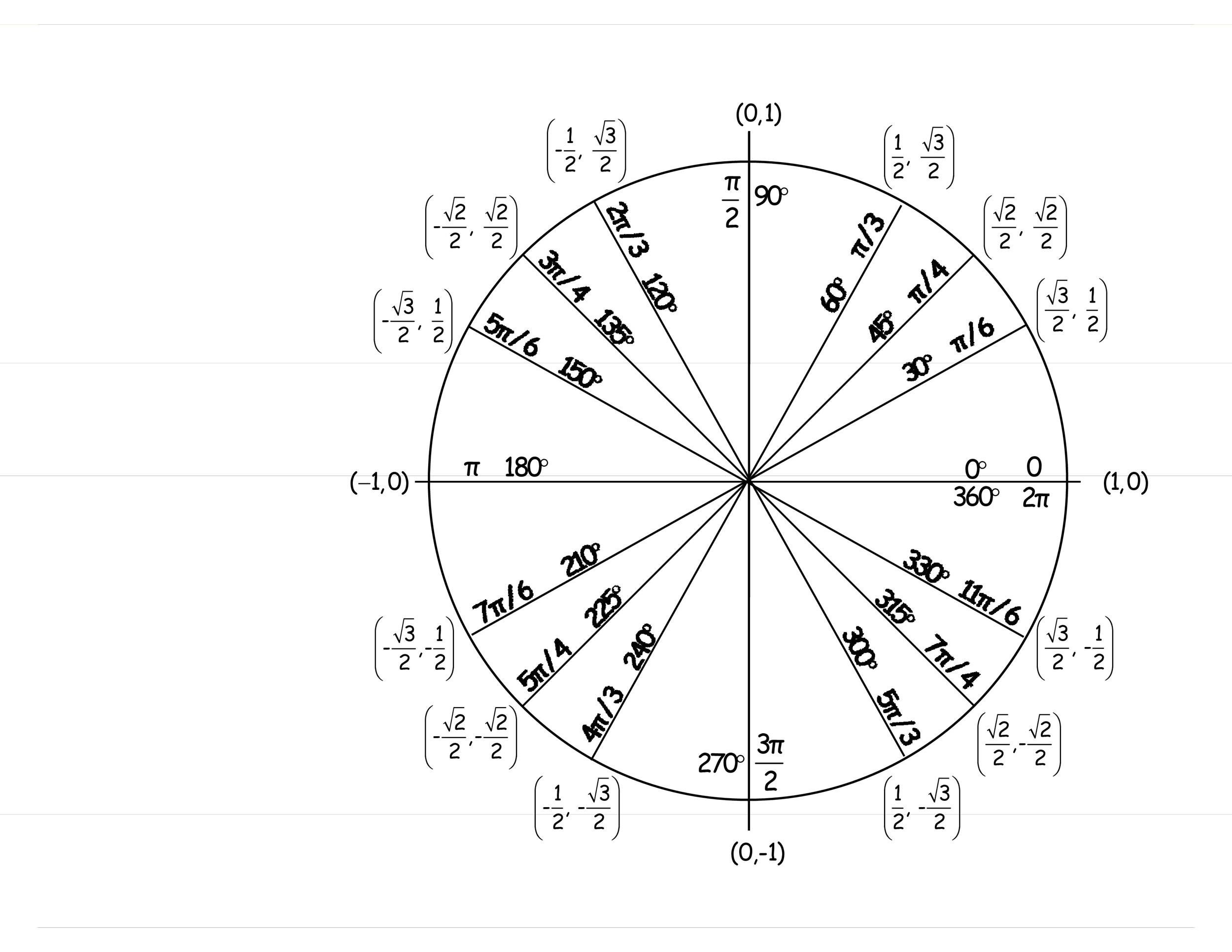



42 Printable Unit Circle Charts Diagrams Sin Cos Tan Cot Etc



Www Berkeleycitycollege Edu Wp Wjeh Files 12 01 Trig Note Circle Approach Pdf




Calculate The Value Of Tan Pi 2 Pi 6 Brainly In
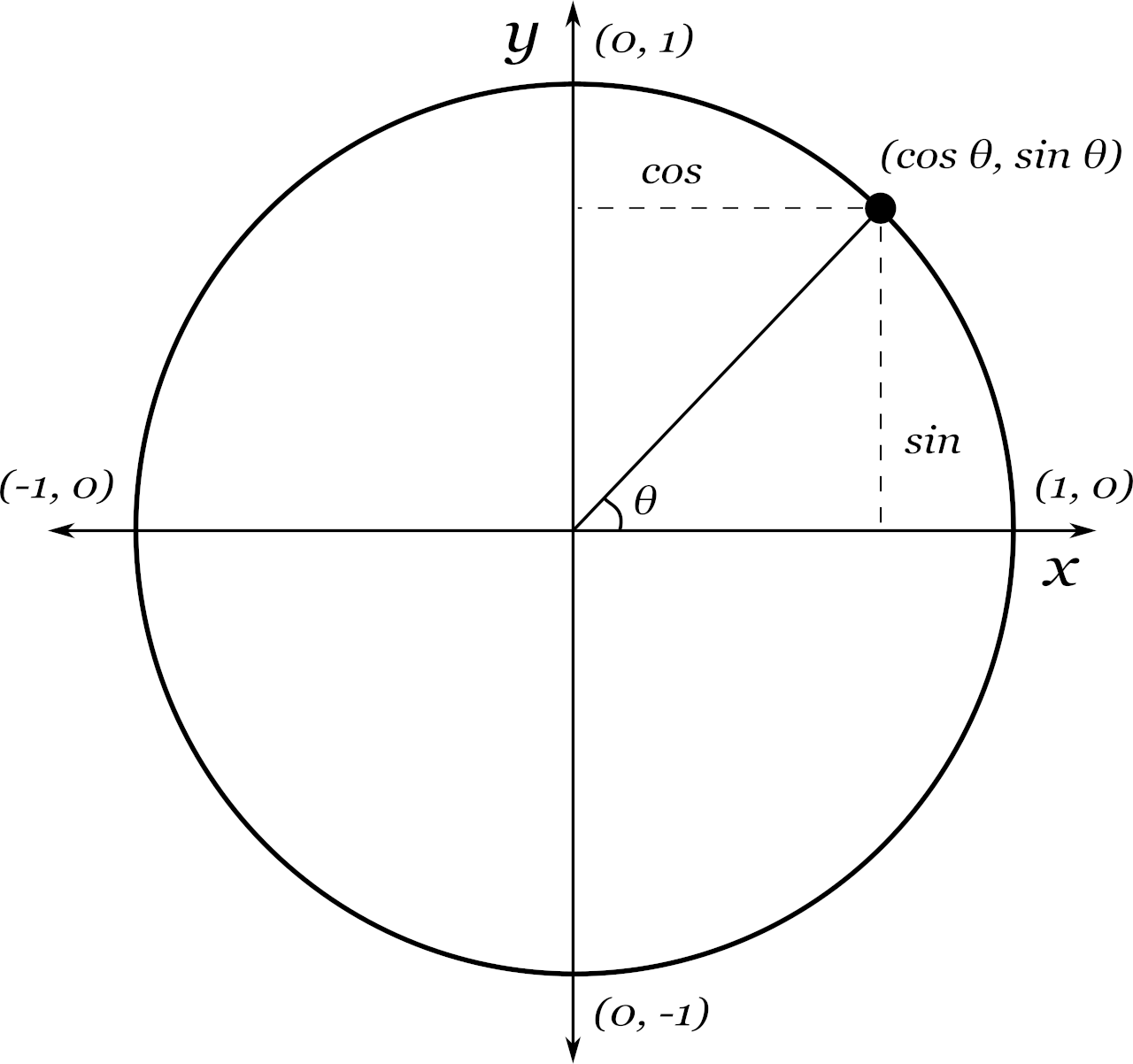



Unit Circle Calculator Inch Calculator




Mfg Inverse Trigonometric Functions



How To Prove That Tan Pi 4 1 2 Arccos A B Tan Pi 4 1 2 Arccos A B 2b A Mathjax Equation In The Details Quora
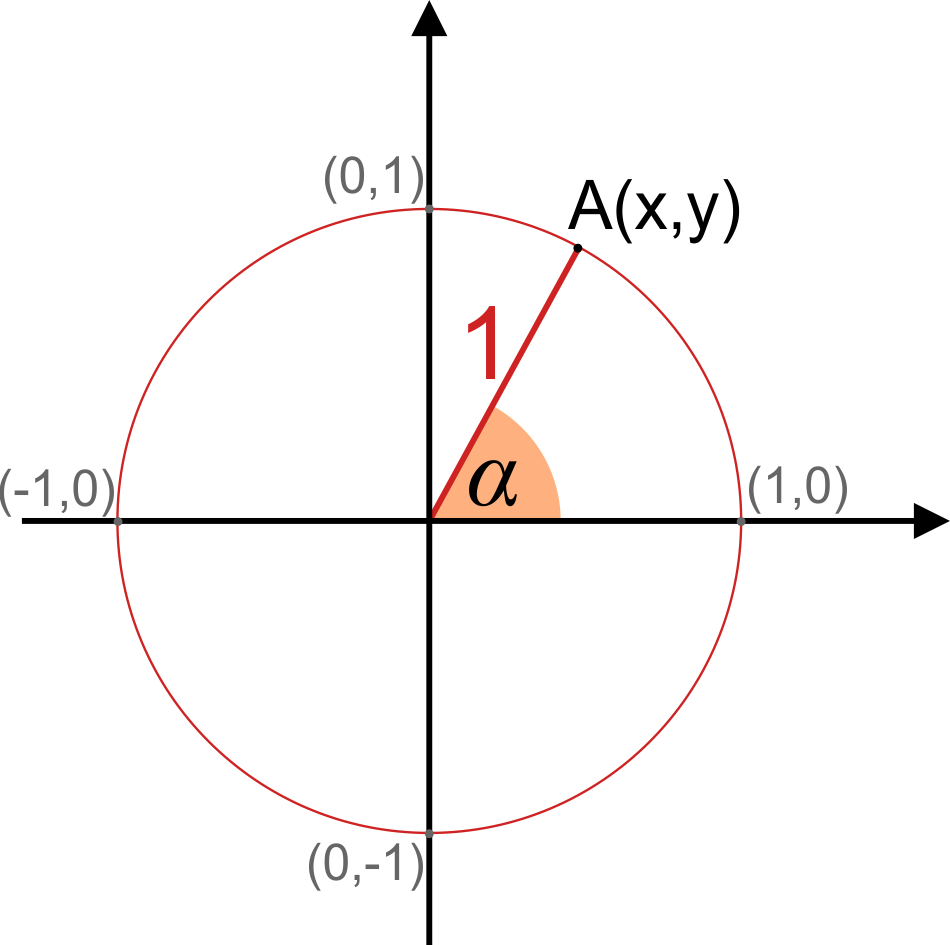



Unit Circle Calculator Find Sin Cos Tan




Cofunction Identities In Trigonometry With Proof And Examples Owlcation
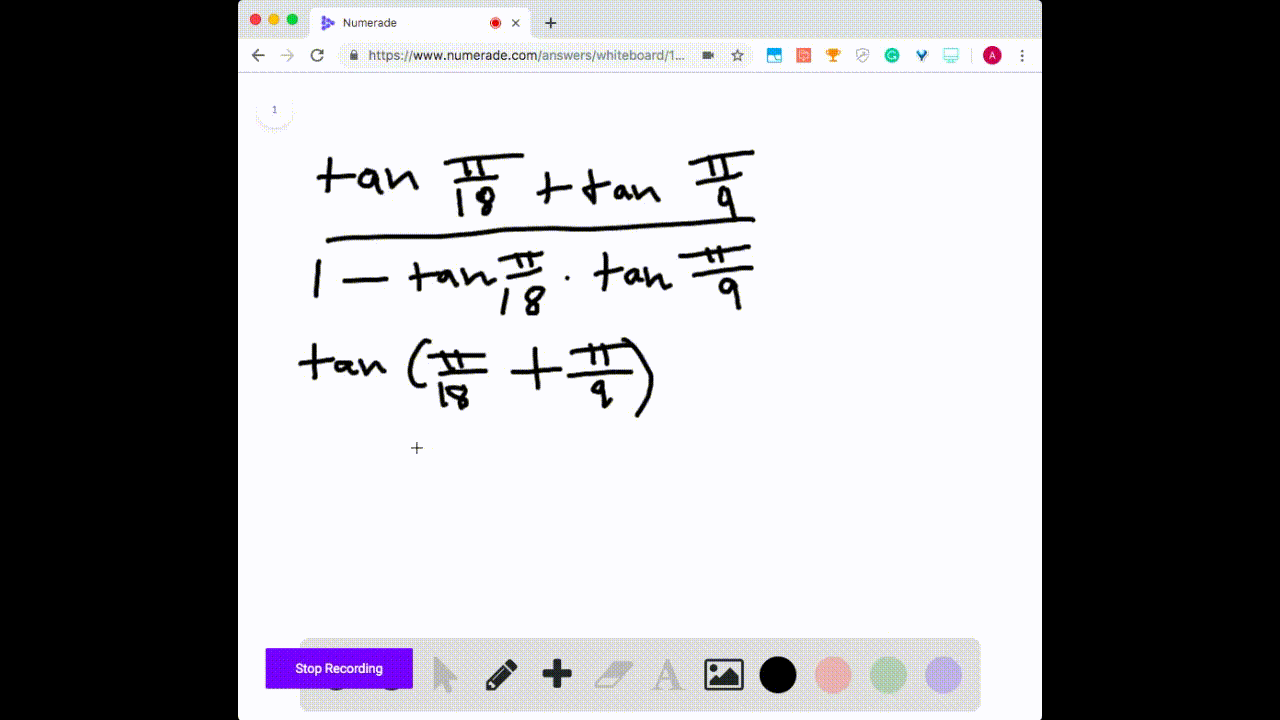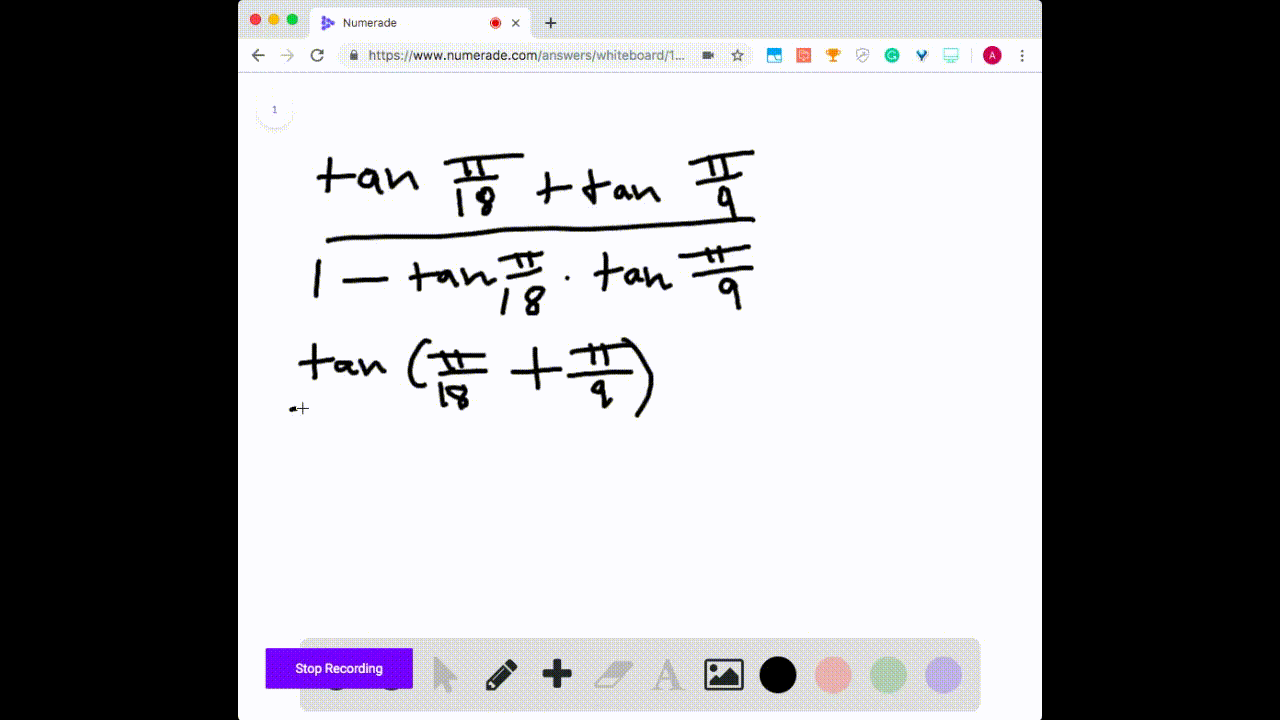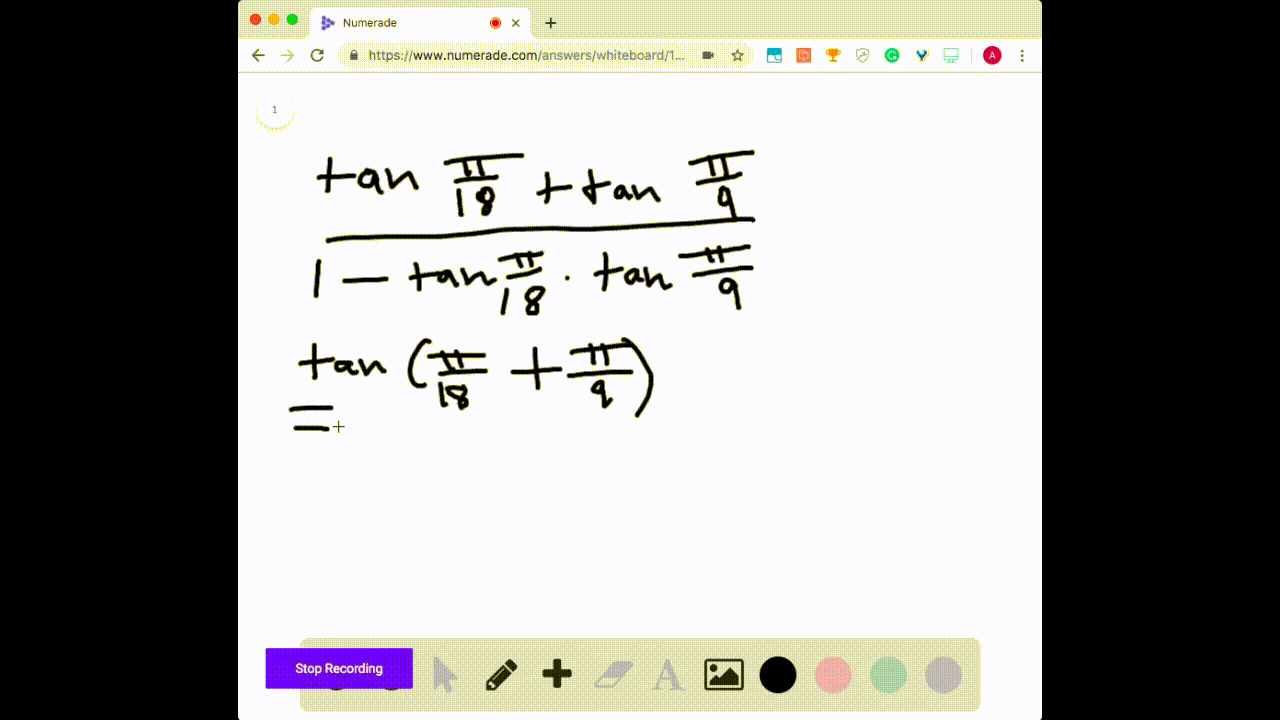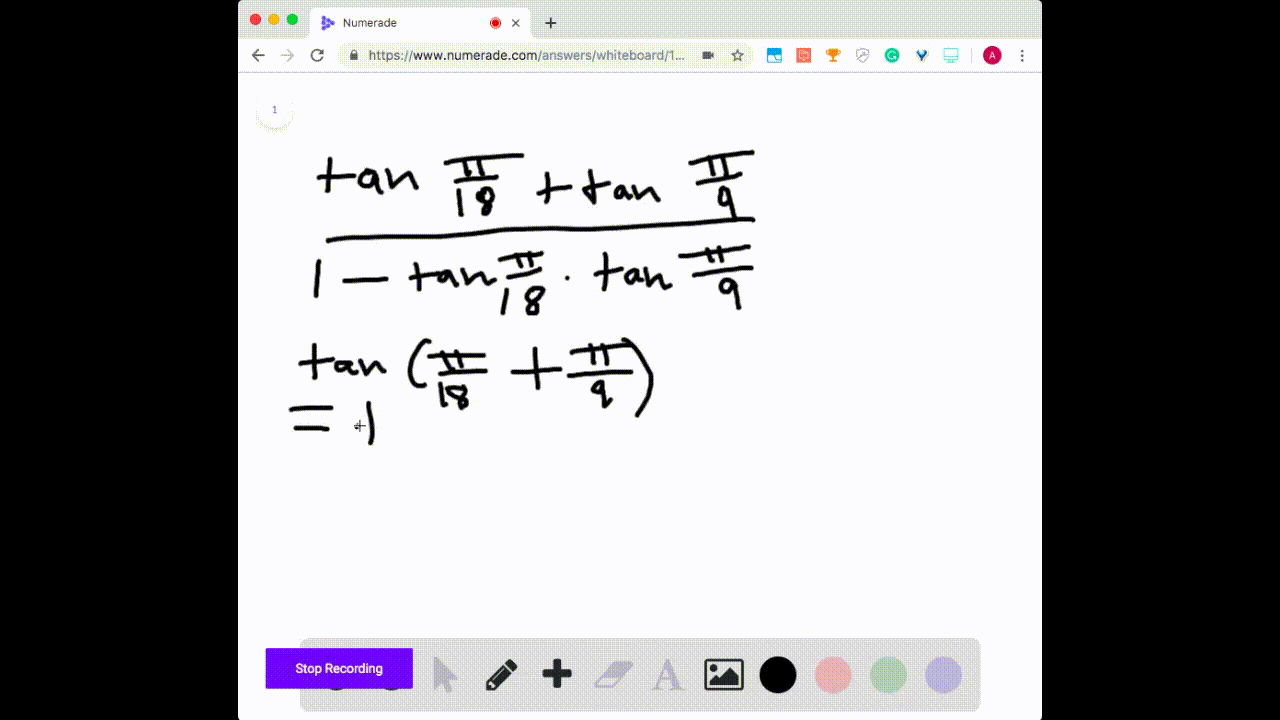



Solved Values Of Trigonometric Functions Use An A
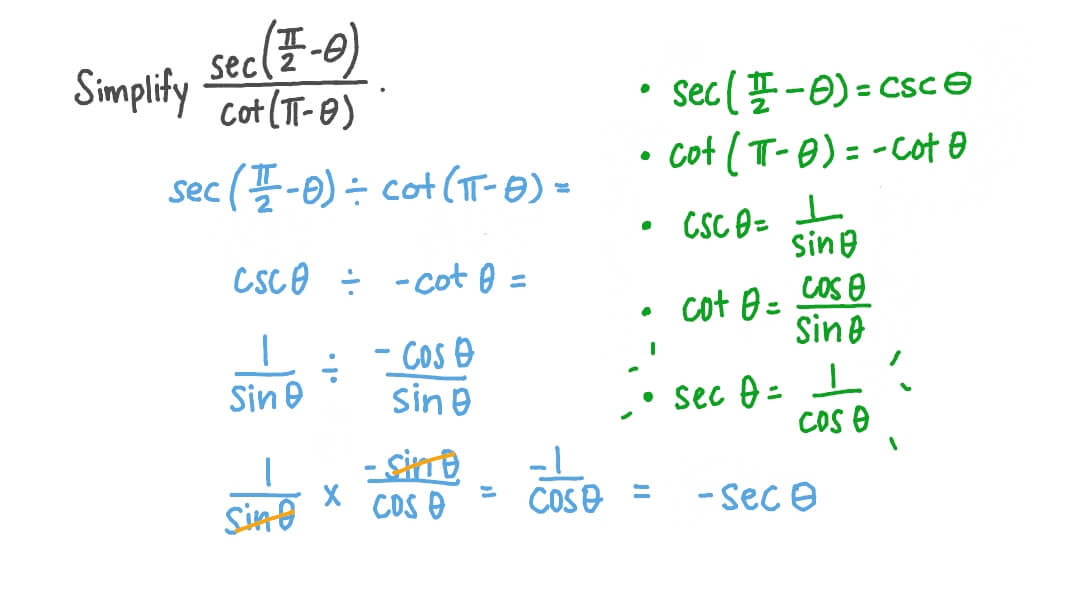



Cos Pi 2
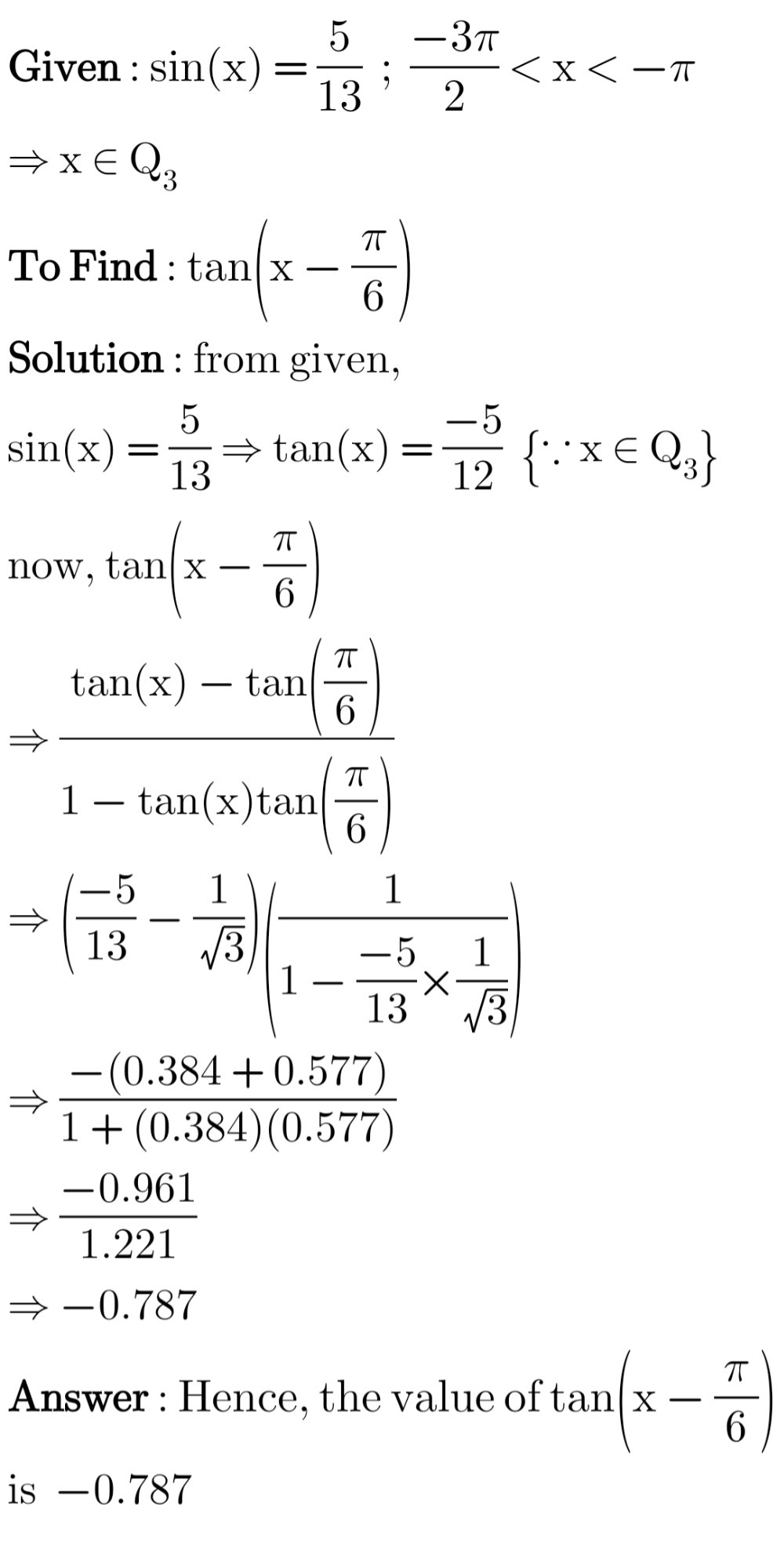



Find The Exact Value Of Tan X Frac P 6 If Sin X Gauthmath




What Are Values Of Trigonometric Ratios For 0 30 45 60 And 90 Degrees




5 Given That Tan 6 31 P 2 Find The Exact Value Chegg Com




Graphs Of The Other Trigonometric Functions Precalculus Ii



Http Cortez Guhsdaz Org Common Pages Displayfile Aspx Itemid
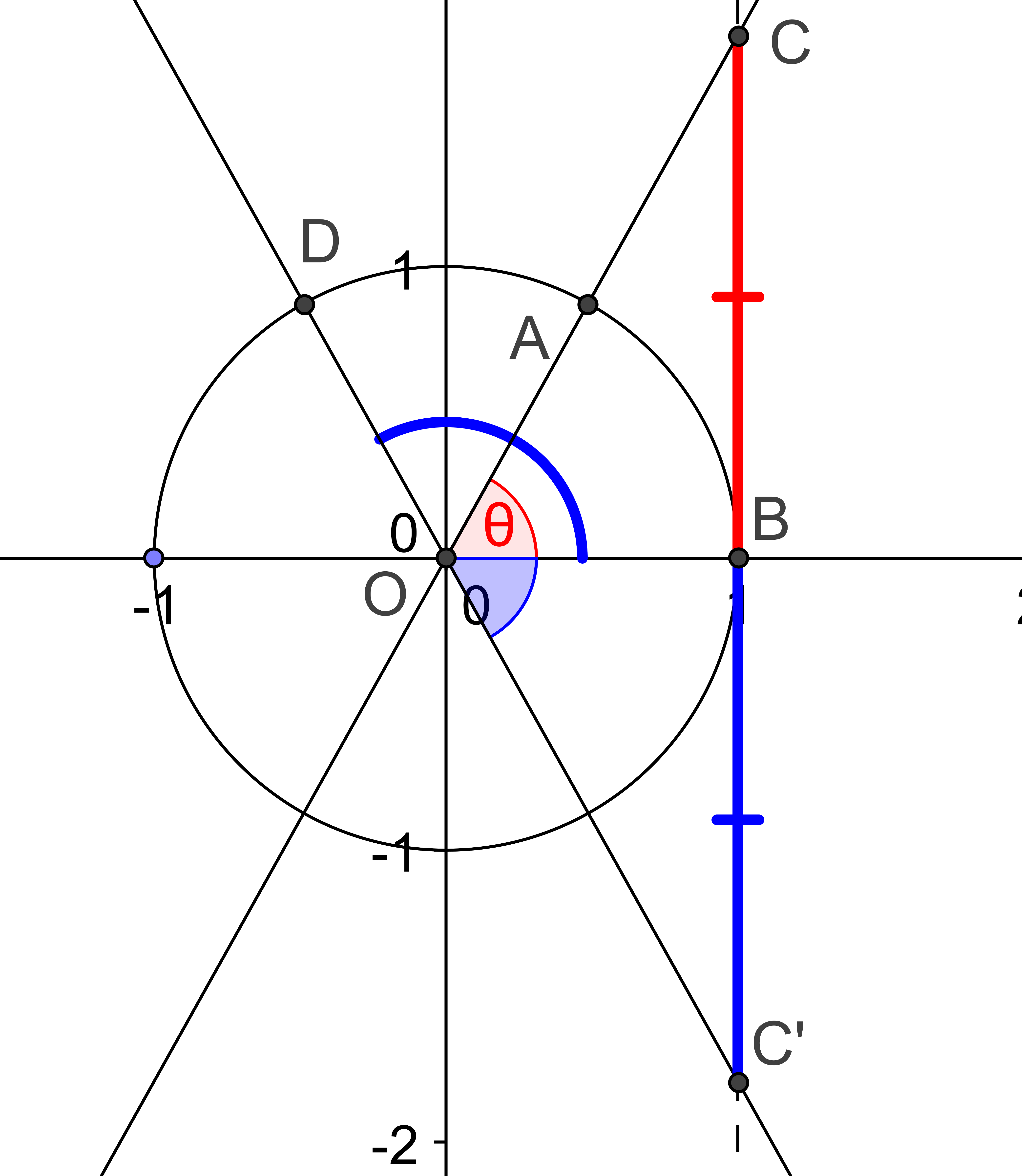



The Tangent Is A Tangent Out Of The Math Box




Prove That Cos X 1 Sin X Tan Pi 4 X 2 Brainly In



Pplato Flap Math 1 6 Trigonometric Functions
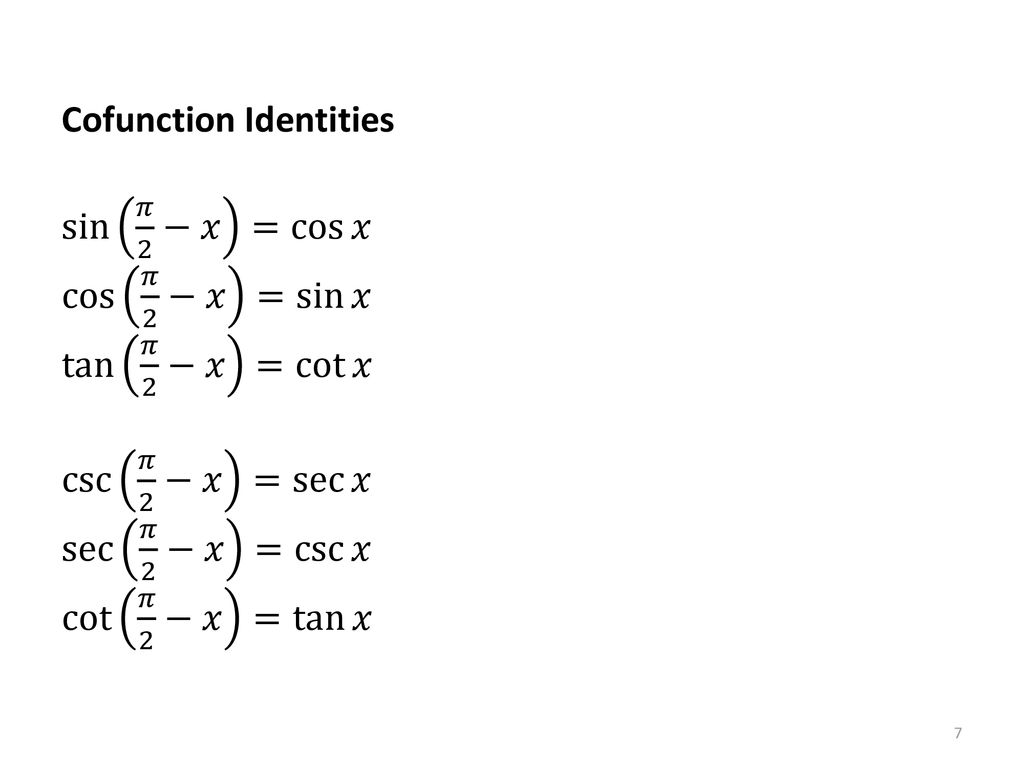



Packet 21 Trigonometric Identities Ppt Download




Find X If Tan 1 4 Cot 1 X Pi 2 Maths Inverse Trigonometric Functions Meritnation Com




Atan2 Wikipedia




Why Is Tan 1 2 Pi Undefined Mathematics Stack Exchange



Http Www Amphi Com Cms Lib010 Az Centricity Domain 256 11 5b half Angle identities Pdf
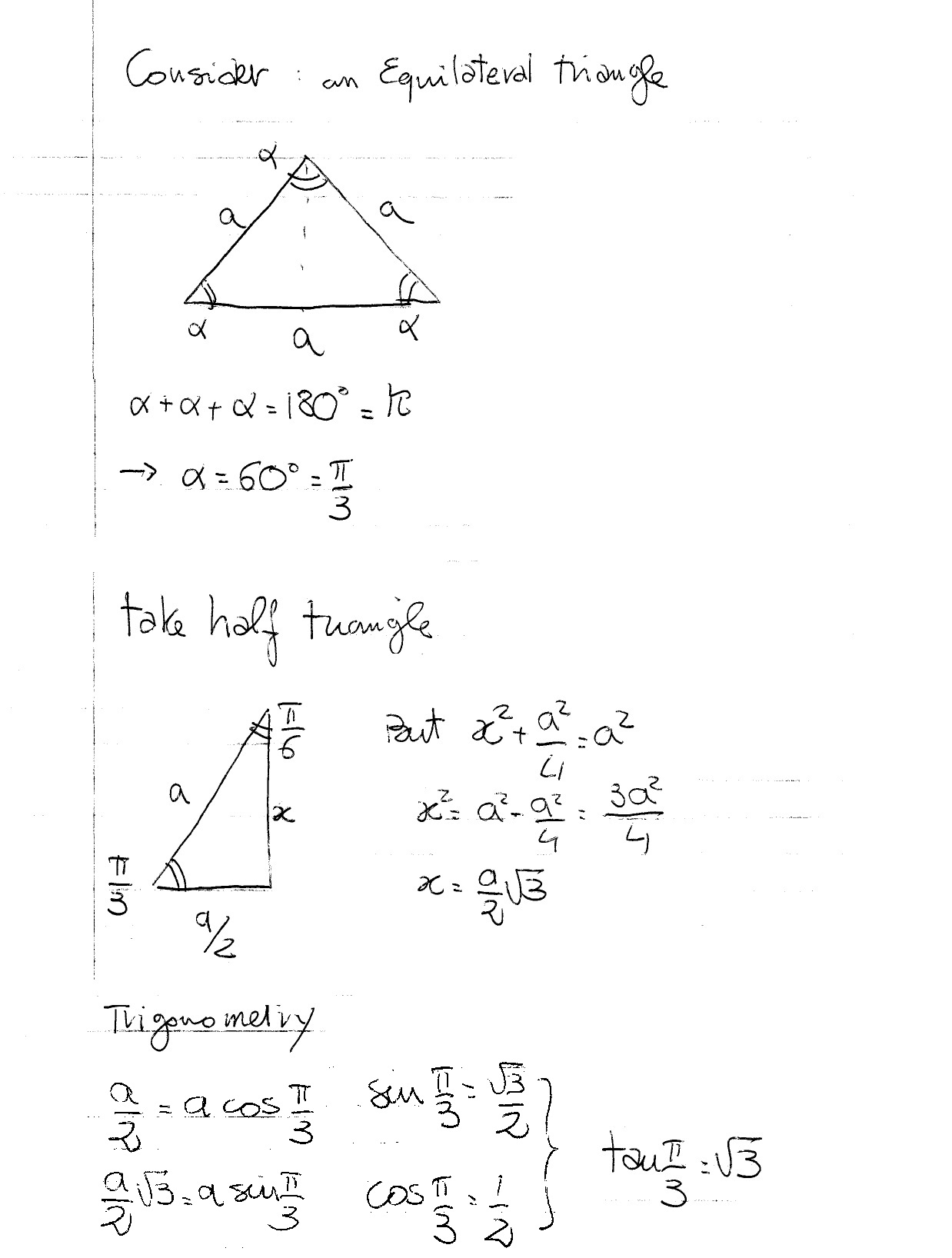



What Is The Value Of Tan Pi 3 Socratic



1
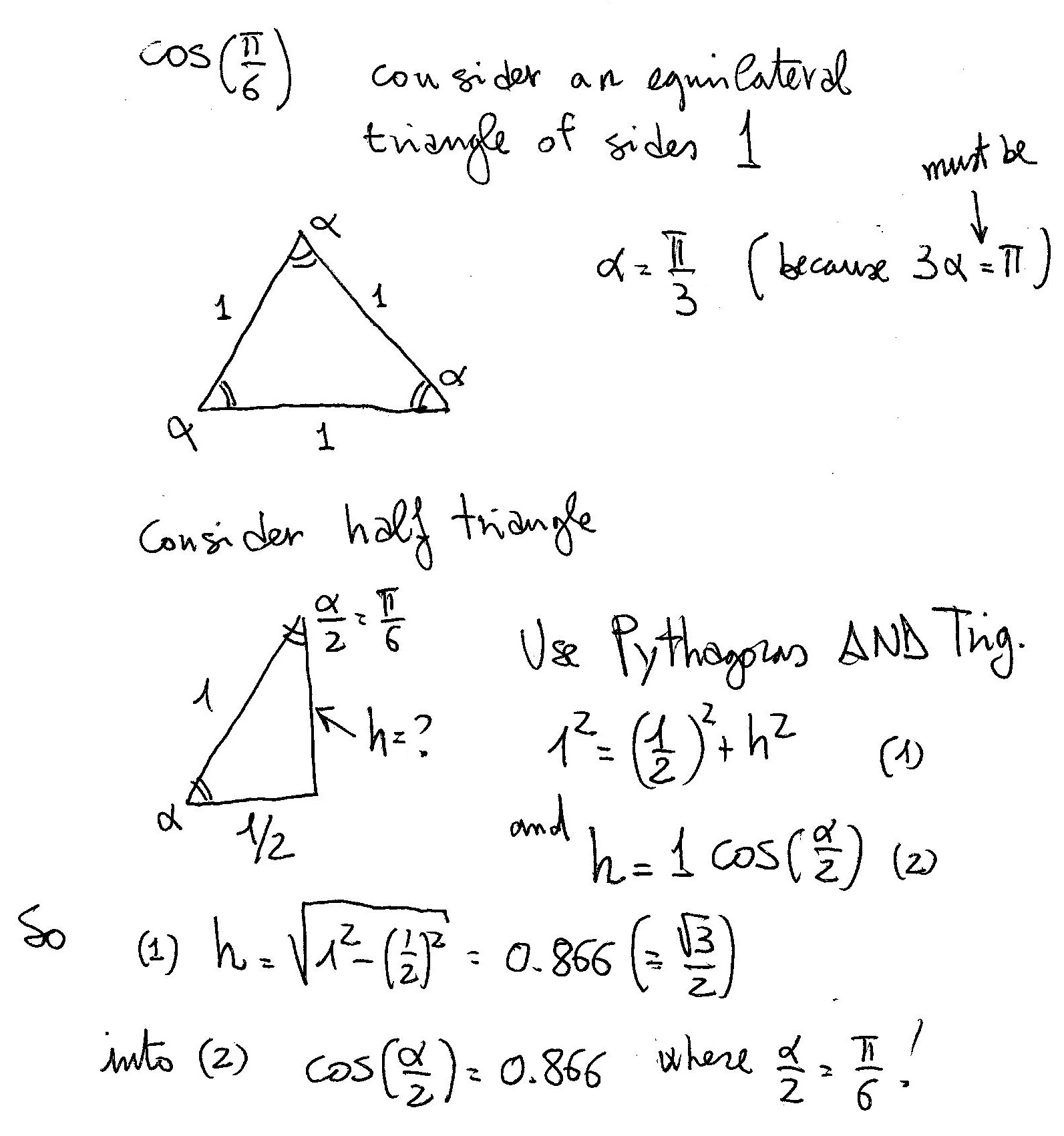



How Do You Find The Exact Value Of Cos Pi 6 Socratic




Determine The Value Of Sin T And Cos T I M Confused On How To Do This Askmath



Efisd Net Common Pages Displayfile Aspx Itemid




Tan Theta Tan Theta Pi 3 Tan Theta Pi 3 Ktan3theta Find K Askiitians



1




Example 27 Find Value Of Tan Pi 8 Chapter 3 Class 11




Cos Pi 2



No comments:
Post a Comment